Consider a free particle of mass m in one dimension.Calculate it's propagator in momentum space:.This propagator is the probability amplitude for a system originally prepared to be in state | Pa> at t=ta to be found in state | Pb> at a later time t=t

来源：学生作业帮助网 编辑：作业帮 时间：2024/11/29 06:38:30
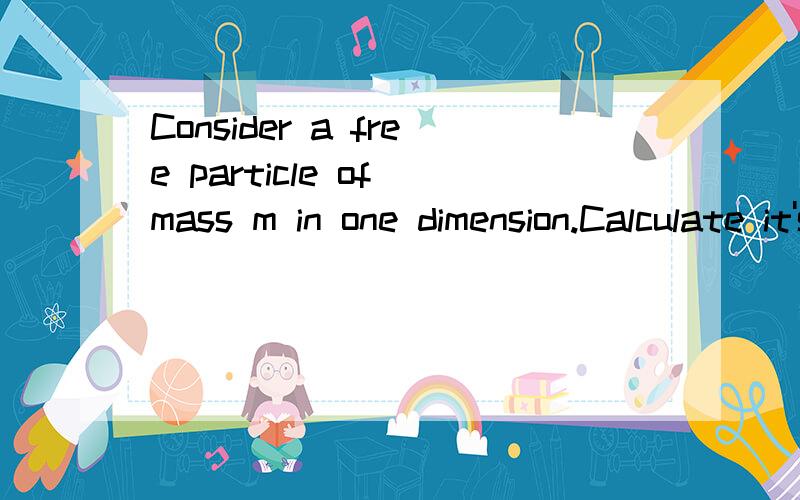
Consider a free particle of mass m in one dimension.Calculate it's propagator in momentum space:.This propagator is the probability amplitude for a system originally prepared to be in state | Pa> at t=ta to be found in state | Pb> at a later time t=t
x=n@ 6XKMn.؂Q  r&wI#m6eHy|<:%@DL C;R65Z3ZUBJHuA1䈆۟D2@Ŗ-ǎ3PZN 6U\C÷_v}7kfS v̜;UՅ\E6Q8>-M=T?`_jPyK,'he7Jy`3#Ys/[m*iZ/ks ÒʎJŜ0%A89JV)&UJ웲Jy

Consider a free particle of mass m in one dimension.Calculate it's propagator in momentum space:.This propagator is the probability amplitude for a system originally prepared to be in state | Pa> at t=ta to be found in state | Pb> at a later time t=t
Consider a free particle of mass m in one dimension.
Calculate it's propagator in momentum space:.This propagator is the probability amplitude for a system originally prepared to be in state | Pa> at t=ta to be found in state | Pb> at a later time t=tb.

Consider a free particle of mass m in one dimension.Calculate it's propagator in momentum space:.This propagator is the probability amplitude for a system originally prepared to be in state | Pa> at t=ta to be found in state | Pb> at a later time t=t
|a,t>=exp[(-iH(t-t0))/h] |a,t0>
we defined K(p'',t;p',t0) satisfying φ(p'',t)=∫dp' {Kφ(p',t0)} //φ(p'',t):=
we can show:K= is a eigenfunction of Hamiltonian which means it can be a stationary state.
and the position of that state should have infinete uncertainly accounting for uncertainly principle.

Ideas to consider could be a weekend get-a-way to your part of the country. I want to find a part-time job.Free time beyond (monday,friday),moral,legal. He often ___(take) a part-time job in his free time. I'll get a part-time job if I have free 1.I'll __get a part-time job _____if I have free time ____ ___ you ___if you have free time.对划线部分提问 英语中consider的用法Both men and woman consider marriage a very important part of their lives.总是习惯性写成”.marriage is a very.错误加了be 动词 那为什么是这样?语法是什么? consider FREE SIGN UP OFFER是什么意思?FREE SIGN UP OFFER: Sign up with NelsonRating to take part in HIGH quality rating for FREE and earn 3000 Points from Pointsmoney.com as a special bonus MAKING MONEY WAS NEVER THAT EASY! 谢谢 But they also 27 beyond the individual places and consider the earth as a 28 .27.[A] pass [B] go [C] reach [D] set 　　28.[A] whole [B] unit [C] part [D] total28题是不是选A,请翻译一下, are you free this afternoon?The underlined part means ___ 英语翻译Still in the tradition of Emerson,Maslow,the phychologist,sees in a new humanism a way to self-actualization.We cannot depend on an objective,value free science to consider important matters. Consider the following words and answer the questions below:a) finger b) disgracefulc) stepsisterd) psycholinguisticse) antidisestablishmentarianismi.Tell the number of morphemes in each word.ii.Underline the free morphemes in each word where possibl 英语翻译1.6 seasons you should consider being a cat2.free rent3.sleep as long as you want4.look great with no effort5.toes look like beans6.license to kill I want to find a job for my free time=I would like to find _____ ______ ______.为什么是part-time job?与for my free time The local government should consider the requests of the residents____parks in the city should be .The local government should consider the requests of the residents____parks in the city should be open to the public free of charge.A.that B.when play a part 还是take a part? 英语翻译The above papers and most other recent work on bank capital regulation assume perfectlyelastic supplies of assets and demands for liabilities on the part of the public.In contrast,Hellmann et al.(2000) consider a setting with monopolistic Don’t you consider it essential enough practice before he takes part in the competition?A.how he will receive B.that he be receivedC.if he will receive D.that he receive Give a free for